Stock News & Analysis

Down 44%, Is Now a Good Time to Buy the Dip in This Warren Buffett Streaming Stock?
Editors’ Picks
HOW THE MOTLEY FOOL CAN HELP YOU
-
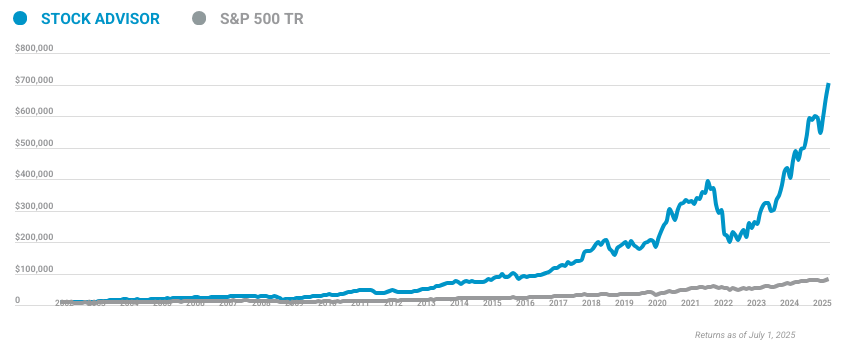
Premium Investing Guidance
Market beating stocks from our award-winning service
-
The Daily Upside Newsletter
Investment news and high-quality insights delivered straight to your inbox
-
Get Started Investing
You can do it. Successful investing in just a few steps
-
Win at Retirement
Secrets and strategies for the post-work life you want.
-
Find a Broker
Find the right brokerage account for you.
-
Listen to our Podcasts
Hear our experts take on stocks, the market, and how to invest.
Premium Investing Services
Invest better with The Motley Fool. Get stock recommendations, portfolio guidance, and more from The Motley Fool's premium services.