Bonds are a more complex investment than common stocks as their prices are immediately impacted by things like inflation or general interest rates in addition to business performance. This makes bond pricing a particularly difficult concept for investors to understand. But if you follow along with some examples in the article below, you'll be able to calculate bond prices like a pro in just 10 minutes.
Starting out with Finance 101
Let's begin with a purely hypothetical question: Suppose I offer to give you $100 in one year. How much is this promise worth?
$100? $90?
There isn't a correct answer. It all depends on the return you can earn in one year's time.
If you want to earn a 25% return in one year, then my promise is only worth $80 ($100/1.25) today. If you want to earn a 50% return, you should value my promise at $66.67 ($100/1.5).
A simple formula allows us to value a dollar in the future based on the return we want on our money. That formula is below.
PV = FV/((1+Rate)^N)
- PV: Present value (the solution -- the value of a future cash flow today).
- FV: Future value (the cash flow you expect to receive in the future).
- Rate: The rate of return you want on your money (expressed as a decimal, so 8% should be entered as 0.08).
- N: Number of periods between now and when you will receive the future cash flow.
Using this formula, we can determine what we should pay for future dollars based on our return requirement and how long we have to wait. If we want to value $100 we expect to receive five years from now, and we require a 10% return, we could calculate the present value by inserting the variables into the formula above:
PV = $100/((1+.10)^5)
Solution: $62.09.
In other words, to get a 10% annual return on our investment, we can only pay $62.09 today in exchange for $100 that we'll receive in five years.
I've created a table showing the value of $100 at various rates of return and time periods. Try working out a few of these on your own and comparing your answers to the table. (If your answer is off by only a penny, it's due to rounding differences in our calculators.)

Bringing the logic to bond pricing
Once you understand the idea of discounting future dollars to the present, the concept of bond pricing is easy to understand. A bond is nothing more than a promise by a government or business to pay you a series of cash flows over time. Thus, we can value each cash flow in present dollars, add the present values up, and the result will be the bond's price -- what it is worth.

Image source: Getty Images.
Let's work through a simple example. Suppose you want to value a bond with a face value of $1,000 that will pay a coupon of $60 semi-annually. It will mature two years after you purchase it. How much is this bond worth?
We first have to identify the cash flows we expect to receive from the bond. We know from the information above that we will receive $60 every six months, plus the par value of $1,000 in two years. Thus, the series of cash flows would look like this:
- Time 0: We pay an unknown amount for this bond (we don't yet know what it is worth!).
- Month 6: We receive $60 in interest.
- Month 12: We receive $60 in interest.
- Month 18: We receive $60 in interest.
- Month 24: We receive $60 in interest plus $1,000 in face value.
We can calculate a present value for each of these cash flows based on our formula, then sum up the results. Let's suppose other bonds with similar risk profiles are paying 10%, which we divide into 5% per semi-annual period.
- The first cash flow is worth $57.14 today, using the formula PV = $60/(1.05^1)
- The second cash flow is worth $54.42 today, using the formula PV = $60/(1.05^2)
- The third cash flow is worth $51.83 today, using the formula PV = $60/(1.05^3)
- The fourth and final cash flow is worth $872.06 today, using the formula PV = $1,060/(1.05^4)
When all the present values are summed, you will find that the bond is worth $1,035.45 based on a 10% return requirement.
Moving your required return up or down will have an opposite effect on how much this bond is worth. If I change the required return to 20% per year, I find that an investor should be willing to pay only $873.21 for the same bond. If I lower the required return to 5%, an investor should be willing to pay as much as $1,131.67 for the bond.
Making it a little easier
If you have followed along so far, you've just learned how to price a bond the hard way by calculating and summing the present value of every expected cash flow. Doing it the long way helps the first time around, but as with anything, there is always an easier method.
You can use a simple time value of money calculator to make quick work of bond pricing. The image below shows how I entered the inputs to calculate the value of the example bond I created above.
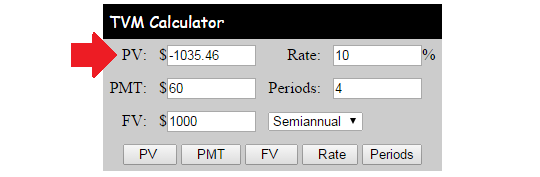
Source: ZenWealth.
Notice the result (PV = -$1,035.46) is just a penny off from what we calculated the hard way, but only because of rounding. The answer is negative because in finance, negative numbers show the direction of money -- it implies that $1,035.46 is what we should pay for the bond.
Playing around with a time value of money calculator will help you understand many key concepts in bonds and finance as a whole. For instance, it's often said that bond prices go down when rates go up. When rates go up, a bond's value should go down, since investors will start using a higher required return to value the bond.
Likewise, we know that bonds which mature further into the future are more susceptible to interest rate changes, since the biggest cash flow (the return of par value of $1,000 at maturity) is discounted by more time periods than a shorter-term bond. The reality is that $1,000 in 30 years is not as valuable as $1,000 next year, nor is it as valuable as $1,000 in your hand right now.
Finally, this very same logic is used to value all kinds of investments, even stocks. After all, like a bond, the value of a business should be equal to the present value of all the money it can pay its owners over its lifetime.