What is it?
The time value of money (TVM) is a basic financial principle describing how money in the present is worth more than an equal amount in the future. As the old saying goes, "A dollar today is worth more than a dollar tomorrow."
Let's say you are the lucky winner of a $1 million lottery, and you are presented with two options: A lump sum payment right now worth $1 million or 10 annual payments of $100,000 each (totaling $1 million over that span of time). Excluding any tax ramifications, which is the better option?
TVM tells us option one -- the lump sum payment right now -- is best because it gives you the ability to put the money to work earning interest or growing via some other investment vehicle like stocks or real estate.
Simple Interest
Why is the time value of money important?
Why is the time value of money important?
Whether you are managing your own finances or determining your investing strategy, TVM is an important concept to comprehend. One critical factor is inflation -- the effect that causes everything to rise in price over time. A McDonald's (MCD -0.26%) hamburger cost just $0.15 back in 1970. Fast-forward 50 years, a burger is going to cost a buck or two, depending on where you live. Your $0.15 was worth much more half a century ago than it's worth today. But what can counteract the negative impacts of inflation? Investing.
Compound Interest
TVM could help your money keep up with -- even exceed -- the rate of inflation, since interest and investment returns compound in value over time. Let's say you earn $1 on $100 in your high-yield savings account (yielding 1% a year). Next year you will earn $1.01 because the first $1 in interest you made is now also earning interest. This same concept works in investing. If your $100 gains 10% in year one (boosting your portfolio value to $110), you will earn $11 in year two if you gain another 10% (since the first $10 made will also gain 10% in value). That might not sound like much in the early stages, but compounding really adds up.
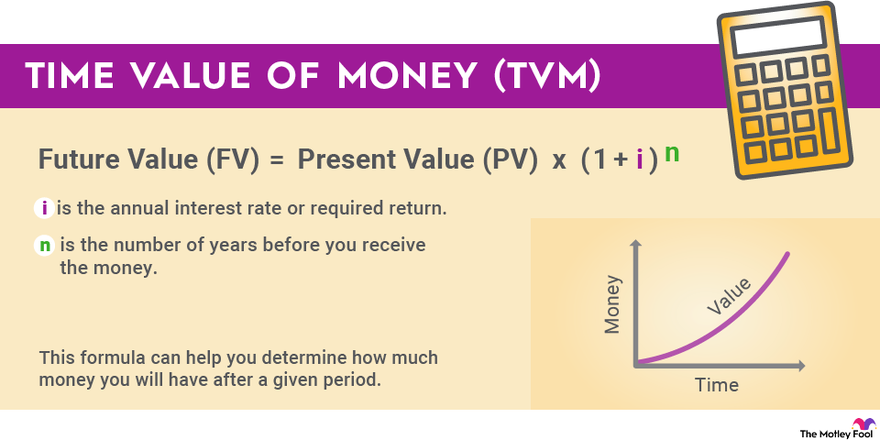
The formula for the time value of money
The formula for the time value of money
The basic formula for TVM is as follows:
This formula can help you determine how much money you will have after a given period. Here is a simple example: Let's say you are purchasing a $1,000 CD from a bank that pays 2% every year. To calculate the value of your money after five years, use this formula:
FV = $1,000 x [ 1 + 0.02 ] ^ (5) = $1,104.08
This formula also illustrates the importance of paying off unsecured debt like credit cards sooner rather than later. Interest payable to the bank compounds in the same way. If you want to save a lot of money, use your dollars now to pay off the loan because the same dollar amount won't be worth as much in the future.
The above equation can also be rearranged to solve for the present value of money based on a future value that is needed:
PV = FV / [ 1 + i ] ^ n
This can be useful if you want to calculate what you think the current fair value of a stock is. Another simplified example: Let's say you think a stock will grow 10% a year for the next five years, at which time you would like to be able to sell each share for $50. If your 10%-per-year projection holds true, what is a fair price to pay for the stock today? You can figure it out as follows:
PV = $50 / [ 1 + 0.10 ] ^ 5 = a current fair value of $31.05 per share
Of course, earning interest on money is much more predictable. Stocks and other equity assets don't provide the same consistent return year in and year out, and in some years even lose value. Over time, though, the average annual return is much higher than for interest-bearing accounts and can help you build wealth faster.
Related Investing Topics
Whether you are budgeting for expenses or planning to save for a future need, TVM is an important concept to understand. It helps with understanding how to combat inflation, why paying off high-interest debt is critical, and how to properly put savings to work (i.e., at the bank or with assets like stocks). TVM is a fundamental principle that can help you make the best decisions to benefit your future self.

























































