Calculating how far a number has declined from one year to the next is pretty easy if you're only considering a one-year period. You subtract the current year's number from the previous year's, divide the result by last year's number, and multiply by 100. If negative, the result is the percentage that metric declined for the year. A positive number reflects an increase.

Red line chart showing downward trend.
Image source: Getty Images.

However, what if you only have data that is two years apart? Or just six months? Or 10 years? Is there a way to calculate the annual percentage change of this data for easy comparison with other annual data?

You bet there is. Here's how.

Breaking down the formula

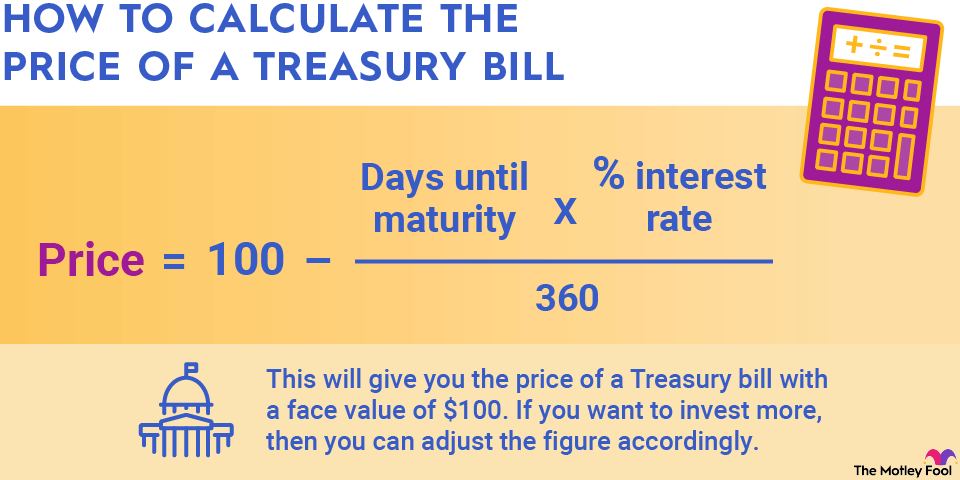
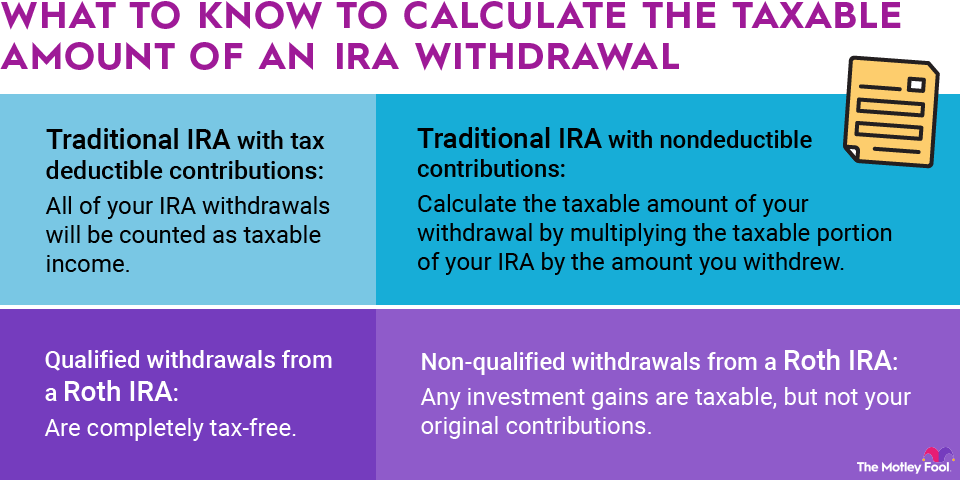
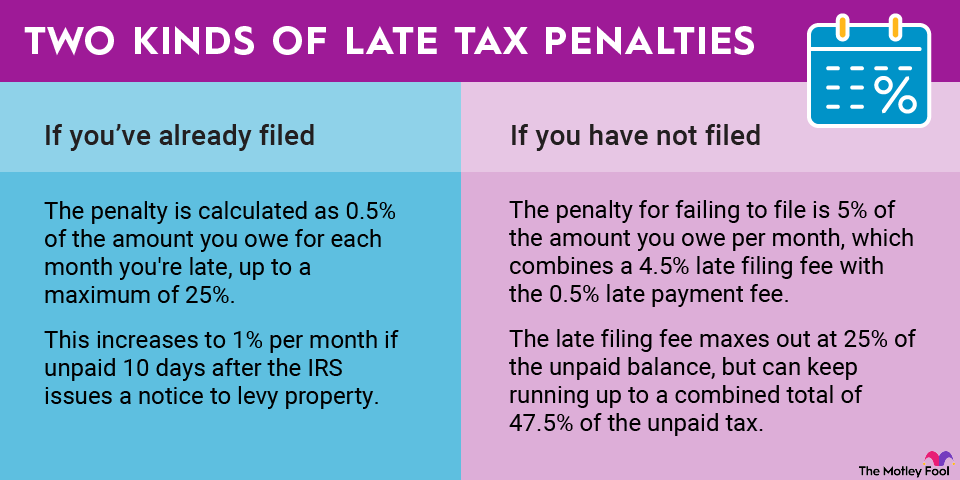
Breaking down the formula

Before we dive into an example, let's first work through the concept. Our first step is to divide the beginning value into the ending value. We do this regardless of how far apart the numbers are in time.

Next, take the number of years between the two numbers and divide that into one. So, if the numbers are two years apart, you would divide one by two years to get 0.5. If the numbers are six months apart (which is 0.5 years), we would divide one by 0.5 years to get 2. Hold onto that number for the next step.

At this point, it's time to pull out your trusty calculator. In this step, we will calculate an exponent using the difference between the two numbers from step one as the base, raised to the power of the time difference divided into one.

To wrap up the calculation, subtract one from this exponent and then multiply by 100 to get the annual percentage change between the two numbers over that period of time. Here's the formula written out altogether:

Formula for annual percent change.

This formula works no matter what metric you're reviewing, whether it's a stock price, a sales number, a profit, or the total assets on a balance sheet.

Sample calculation

Percentage of annual decline formula in action

Let's say a company's revenue declined from $15 million to $10 million over a two-year period. We want to calculate the percentage of annual decline.

First, we divide the most current revenue number by the beginning revenue number: $10 million divided by $15 million comes to 0.667.

Related investing topics

Assets vs. Revenue: What's the Difference?

An explanation of an asset compared with an explanation of revenue with examples of each.

How to Calculate Dividends (With or Without a Balance Sheet)

There's a formula to calculating dividends. Learn how to use it to find yours.

How to Calculate Tax on W-9 Income

Form W-9 doesn't usually result in income taxes withheld, but there is an exception.

How to Calculate Accrued Interest

Learn to calculate accrued interest on loans or credit cards, considering rates, daily balances, and precision methods.

We'll use our calculator to raise 0.667 to the half power. We use half because the formula tells us to divide one by the number of years difference between the two numbers; in this case, that's two years. So, 1.5 raised to the half power gives us 0.816.

An example using the formula for annual percent change.

Finally, we subtract one and multiply by 100 to give us the annual percent decline. In this case, that works out to an 18.4% average annual decline over this two-year period.

Investing in stocks involves a lot of research, starting with who you open your investing account with. Click here to visit our broker center to compare and contrast your options.

The Motley Fool has a disclosure policy.