The discounted cash flow model is used to value companies in the present based on expectations of future cash flows. As the model's name implies, the expected cash flows are discounted back to their values today. The discounted cash flow model -- often abbreviated as the DCF model -- certainly is not a perfect valuation tool, but it does help to give an idea of what a company is worth.
Valuation
In this article, we'll take a look at the discounted cash flow model and how it can be applied to stock valuation, plus an example of how cash flows are discounted to calculate a stock's worth and some of the other valuation methods investors can use to determine the theoretical values of stocks.

How does the discounted cash flow model work?
How does the discounted cash flow model work?
The basic concept underlying the discounted cash flow model is that businesses are theoretically worth the present value of all of their future cash flows. So let's start with the idea of present value. Because money can be invested to generate returns, and inflation generally makes the value of a dollar worth progressively less over time, money today is thought to be worth more than an equivalent amount of money at some point in the future. The further in the future, the higher the discount or the less the money is worth.
For example, if I invest $1,000 today in a Treasury bond with a 2% yield, in a year that $1,000 would be worth $1,020. Conversely, if I know that I'm getting a payment of $1,000 in one year from now, then the present value of that $1,000 is 2% less because it is money that is not already in my portfolio generating returns.
The DCF calculation method
The DCF calculation method
As a fair warning, calculating discounted cash flow valuation isn't exactly a mathematically light process. Fortunately, there are some excellent DCF valuation calculators that will do the hard work for you, but it's still important to understand how the method works. When calculating DCF valuations yourself, be sure to use a DCF calculator that is designed for valuing stocks.
With that in mind, here's a rundown of how DCF calculations work. The general formula for a discounted cash flow valuation analysis is:
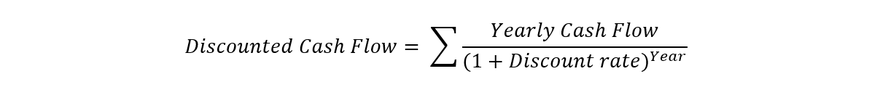
That large symbol at the front of the formula is the Greek letter sigma, and it is used to denote the sum of several quantities. In other words, this symbol tells you to perform a present value calculation for each year's cash flow and then add them all together. Here's what the formula looks like in long form:

The "r" in the formula is the discount rate and the "n" is the number of years in the future. For the discount rate, some analysts use the company's estimated cost of capital, while others use the expected return of a comparable benchmark index like the S&P 500 (SNPINDEX:^GSPC). I'm generally a fan of using the latter.
It's common practice to use earnings per share, or EPS, in place of "cash flow." These don't mean the exact same thing, but for stocks, using the present value of earnings is a reasonable method.
Example stock valuation using the discounted cash flow model
Example stock valuation using the discounted cash flow model
With all of the above in mind, let's take a look at a real-world example. As of this writing, Apple (AAPL 0.33%) stock trades for about $195 per share. Wall Street analysts are forecasting $1.53 in earnings per share in 2024, and the company's earnings are expected to grow by 9% annually over the next five years. For the purpose of this calculation, we'll assume that Apple's annual earnings growth slows to 5% in perpetuity after five years, and that the expected annualized growth rate of the S&P 500 is 10% for the foreseeable future.
Of course, the formula considers all of Apple's future cash flows, which isn't practical to calculate by hand. But just to give you a glimpse of the mathematics behind the method, here are the first few parts of the infinite sum of future discounted cash flows:
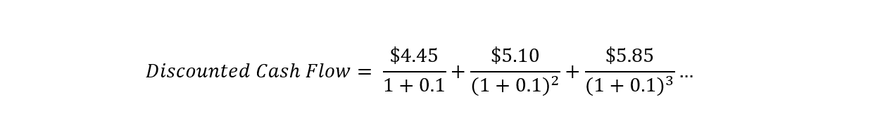
Using a financial calculator produces the sum of the present values of Apple's future cash flows: $140.46.
Other valuation methods to use
Other valuation methods to use
In the end, DCF modeling is just one way to evaluate a stock. And, as mentioned earlier, DCF models are not perfect valuation tools. They don't provide all the answers, but they can give us ideas about what we should pay for our investments.
The right approach might be to use a combination of valuation methods to evaluate stocks. To be clear, none of them are perfect. Unfortunately, there's no such thing as a 100% reliable stock valuation method. If there were, we'd all get rich very quickly and easily. This is certainly not an exhaustive list, but here are some of the other popular valuation methods:
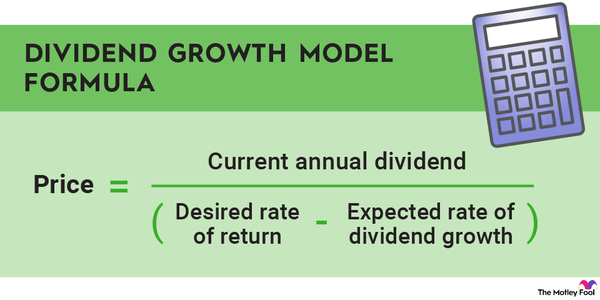
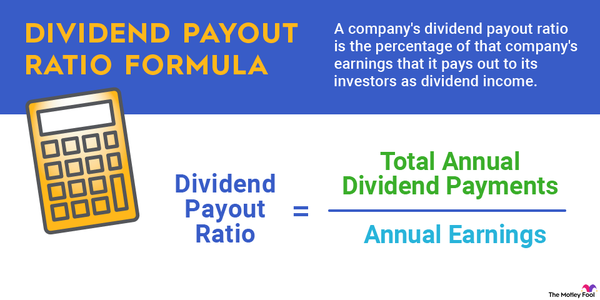
- Dividend discount model: This type of model uses a stock's current dividend rate, estimated future dividend growth, and the company's cost of capital to estimate the stock's theoretical value. Using a dividend discount model can be a smart way to calculate the value of dividend-paying stocks and is especially useful if a stock's dividend is the primary reason you're interested in the stock. But using this valuation method has some obvious and significant shortcomings since the percentages of profits that dividend stocks pay always vary. Future dividend payments or dividend growth is not guaranteed, and the cost of capital can be extremely difficult to accurately predict.
- Residual income model: This type of model values a company based on its estimated future income. Some companies don't have positive cash flows (or earnings) and don't pay dividends, so the discounted cash flow and dividend discount models don't work very well. In these cases, the residual income model is best.
Beyond these options, there is no shortage of other valuation methods, which is why, if you ask 10 different analysts what a particular stock is worth, you're likely to get 10 different answers.
Related investing topics
The bottom line on the DCF model
The bottom line on the DCF model
It may be fairly obvious by now what is the biggest weakness of the DCF valuation method: Look at all the assumptions! You have to assume growth rates, for both earnings and the S&P 500 benchmark index, for the foreseeable future. Neither can be predicted with a high level of accuracy, especially earnings growth (this is why stock prices tend to move sharply in one direction or the other after earnings reports -- because investors are often surprised at the results).
However, the DCF method isn't without its merits. Using it, we can see how close a stock is trading to its assumed fair value. Developing a discounted cash flow model can be a good way to theoretically value a stock investment, but it isn't an exact science. Investors should use DCF modeling as one tool in their analytical toolkits when evaluating prospective investments.